|
Topology Optimization in Structural and Continuum Mechanics |
 |
 |
 |
 George.I.N.Rozvany, Tomasz Lewiński (Eds.) , Topology Optimization in Structural and Continuum Mechanics. CISM International Centre for Mechanical Sciences 549. Courses and Lectures. Springer Wien Heidelberg New York Dordrecht London, CISM, Udine 2014. 471 pp. George.I.N.Rozvany, Tomasz Lewiński (Eds.) , Topology Optimization in Structural and Continuum Mechanics. CISM International Centre for Mechanical Sciences 549. Courses and Lectures. Springer Wien Heidelberg New York Dordrecht London, CISM, Udine 2014. 471 pp.
Artykuły pracowników Zakładu:
G.I.N. Rozvany, T. Sokół: Validation of Numerical Methods by Analytical Benchmarks, and Verification of Exact Solutions by Numerical Methods, pp. 53-69, In: George I. N. Rozvany, Tomasz Lewiński (Eds.), Topology Optimization in Structural and Continuum Mechanics. CISM International Centre for Mechanical Sciences 549. Courses and Lectures. Springer Wien Heidelberg New York Dordrecht London, CISM, Udine 2014.
T.Lewiński, T.Sokół, On basic properties of Michell's structures pp. 87-128. In: George.I.N.Rozvany, Tomasz Lewiński (Eds.), Topology Optimization in Structural and Continuum Mechanics. CISM International Centre for Mechanical Sciences 549. Courses and Lectures. Springer Wien Heidelberg New York Dordrecht London, CISM, Udine 2014.
G.Dzierżanowski, T.Lewiński, Compliance minimization of two-material elastic structures. pp 175-212. In: George.I.N.Rozvany, Tomasz Lewiński (Eds.) , Topology Optimization in Structural and Continuum Mechanics. CISM International Centre for Mechanical Sciences 549. Courses and Lectures. Springer Wien Heidelberg New York Dordrecht London, CISM, Udine 2014.
S.Czarnecki, T.Lewiński, The Free material design in linear elasticity. pp 213-257. In: George.I.N.Rozvany, Tomasz Lewiński (Eds.) , Topology Optimization in Structural and Continuum Mechanics. CISM International Centre for Mechanical Sciences 549. Courses and Lectures. Springer Wien Heidelberg New York Dordrecht London, CISM, Udine 2014.
Read more>> |
|
Topology optimization in structural mechanics |
 |
 |
 |
T opology optimization in structural mechanics opology optimization in structural mechanics
T.Lewiński, S. Czarnecki, G.Dzierżanowski and T. Sokół
BULLETIN OF THE POLISH ACADEMY OF SCIENCES TECHNICAL SCIENCES, Vol. 61, No. 1, 2013
Optimization of structural topology, called briefly: topology optimization, is a relatively new branch of structural optimization. Its aim is to create optimal structures, instead of correcting the dimensions or changing the shapes of initial designs. For being able to create the structure, one should have a possibility to handle the members of zero stiffness or admit the material of singular constitutive properties, i.e. void. In the present paper, four fundamental problems of topology optimization are discussed: Michell's structures, two-material layout problem in light of the relaxation by homogenization theory, optimal shape design and the free material design. Their features are disclosed by presenting results for selected problems concerning the same feasible domain, boundary conditions and applied loading. This discussion provides a short introduction into current topics of topology optimization.
Read more>> |
|
On minimum compliance problems of thin elastic plates of varying thickness |
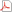 |
 |
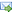 |
 On minimum compliance problems of thin elastic plates of varying thickness On minimum compliance problems of thin elastic plates of varying thickness
S. Czarnecki, T. Lewiński
Structural and Multidisciplinary Optimization
Read online>>
The paper deals with two minimum compliance problems of variable thickness plates subject to an in-plane loading or to a transverse loading. The first of this problem (called also the variable thickness sheet problem) is reduced to the locking material problem in its stress-based setting, thus interrelating the stress-based formulation by Allaire (2002) with the kinematic formulation of Golay and Seppecher (Eur J Mech A Solids 20:631–644, 2001). The second problem concerning the Kirchhoff plates of varying thickness is reduced to a non-convex problem in which the integrand of the minimized functional is the square root of the norm of the density energy expressed in terms of the bending moments. This proves that the problem cannot be interpreted as a problem of equilibrium of a locking material. Both formulations discussed need the numerical treatment in which stresses (bending moments) are the main unknowns. |
|
Exact analytical solutions for some popular benchmark problems in topology optimization III: L-shaped domains revisited |
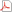 |
 |
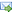 |
 Exact analytical solutions for some popular benchmark problems in topology optimization III: L-shaped domains revisited Exact analytical solutions for some popular benchmark problems in topology optimization III: L-shaped domains revisited
T. Lewiński, G. I. N. Rozvany, T. Sokół, K. Bołbotowski
Structural and Multidisciplinary Optimization
June 2013, Volume 47, Issue 6, pp 937-942
Read online>>
The paper provides the corrections and extensions of the paper of the same title, published in vol. 35, 2008, pp. 165–174 of the current journal. The analytical solutions of Michell trusses constructed within the L-shaped domains are confirmed by the ground structure method of the truss optimization, thus giving the upper bounds of the exact solutions.
|
|
Exact least-volume trusses for two symmetric point loads and unequal permissible stresses in tension and compression |
 |
 |
 |
 Exact least-volume trusses for two symmetric point loads and unequal permissible stresses in tension and compression Tomasz Sokół · George I. N. Rozvany Exact least-volume trusses for two symmetric point loads and unequal permissible stresses in tension and compression Tomasz Sokół · George I. N. Rozvany
Structural and Multidisciplinary Optimization
January 2013, Volume 47, Issue 1, pp 151-155,
Read online>>
In this Authors' Reply previously presented solutions are extended to unequal permissible stresses in tension and compression, comments are made on the lack of adjoint displacement fields in so-called O-regions (memberless regions), and some computational details are explained. |
|
|





 opology optimization in structural mechanics
opology optimization in structural mechanics On minimum compliance problems of thin elastic plates of varying thickness
On minimum compliance problems of thin elastic plates of varying thickness Exact analytical solutions for some popular benchmark problems in topology optimization III: L-shaped domains revisited
Exact analytical solutions for some popular benchmark problems in topology optimization III: L-shaped domains revisited
